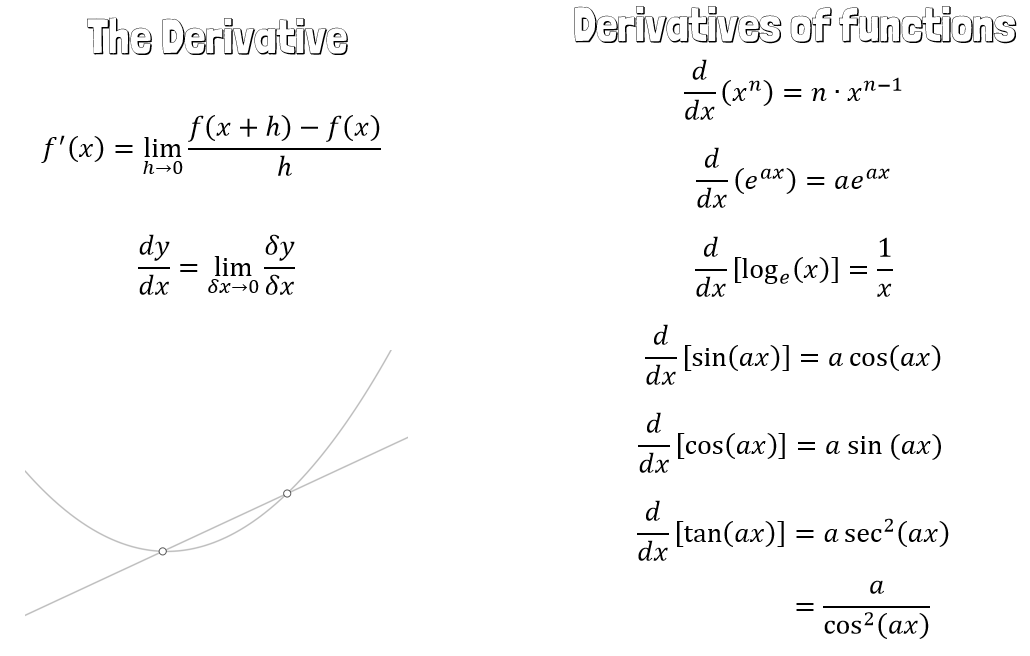
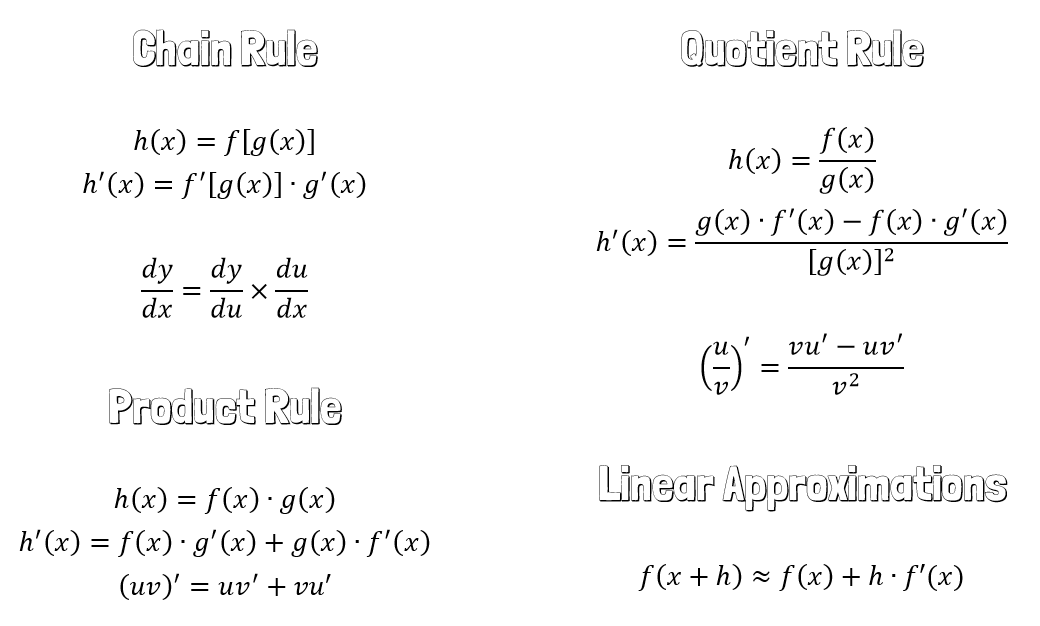This one’s going to be mostly about ICT, but I promise I do tie it into maths at a few points. In my defence, the tagline to the blog is “maths and stuff“.
I created a new ICT subject this year. I guess technically I created it couple of years ago, but that was really just a name and a short description. First semester was the first time I had to actually plan and deliver it.
The subject was “ICT: Web development”, a semester long elective subject for Year 9 & 10. There’s been a gap in our school’s ICT offerings for a while. We have compulsory ICT up to Year 8, and I teach VCE IT*. Which is weird: we keep getting kids choosing IT in Year 11 without choosing the ICT electives in the two years prior. The existing ICT subjects were poorly defined and didn’t link that well into VCE (and from what I gather, involved playing “Zoo Tycoon” a lot in the past). So the P-8 ICT teacher and I discussed how we can improve the subjects and came up with two new ones, to alternate each year: “ICT: Web Development” and “ICT: Software”.
So how did it go? Well…
I saw some great successes, as well as a lot of things I need to work on for next time.
One benefit was the fact that this was an elective, so of course everyone was intrinsically motivated in it from the start, right? OK, not really. But even if I hadn’t stated it as such at the start of the year, I think deep down that was an assumption I was making. That’s not to say there weren’t highly motivated students; some were very motivated, keen to build their own creative websites and learn what they could about how the internet works. But in some of the others I think I mistook enthusiasm for playing around with computers for enthusiasm for the subject matter.
As an aside, I think that’s an easy mistake to make in any subject, especially maths. This has been stated a million times before, but I’ll say it again: we need to ensure that technology is used in a way that supports learning, not because “it will be fun”.
I basically split the semester in two parts – first term was learning basic web development concepts such as HTML, CSS and a little JavaScript, as well as introducing the fun sounding ‘Problem-solving Methodology’. (This is a compulsory part of VCE IT, so if any of these students pick up IT in Year 11, they’ll have a head start on understanding a lot of theory. It basically describes four steps in producing IT solutions: Analysis, Design, Development and Evaluation.)
The second term was focussed on a major project of the students’ own choice. Some of them worked on projects for real clients (usually businesses or organisations run by their parents), and some produced “fan sites” about things they were interested in.
I gave them a choice of working in groups or individually. I don’t know what I’ll do in the future. I was hoping that the groups would find ways to divide up the workload, and some managed to do this well. Unfortunately the groups found it difficult to communicate decisions clearly, and often forgot to share all their work with each other. One group successfully used a shared folder on Dropbox to collaborate – they also shared the folder with me so I could see their work and help them quickly if they needed it. Then with another group, a student spent a whole lesson looking for an email account they remembered the password to so they could sign up to Dropbox.
I’m not against kids working in groups, but I’m not sure it works as well for projects as large as this. Some showed it can work, but I think others didn’t produce projects as impressive as they would have by themselves.
Next time I’ll need to spend more time encouraging kids to experiment with their work. Working with code can be a scary prospect when it’s new, and students reacted differently to it. Some dove in head first, willing to try different things and see what the results were. The quality of the feedback computers provide to students in other subjects is somewhat dubious, but they are fantastic at providing feedback when computers themselves are the subject.
Some students would try different methods in their code, and when their website didn’t work as they expected, they tried something else. When they ran into problems they couldn’t fix, they could ask me specific questions about how to deal with it.
But other students weren’t willing to experiment, and would only write code when they new exactly the way they were supposed to do it. When these students asked for help, they often phrased their question as “My website doesn’t work.” As a result of their unwillingness to take risks, their understanding developed a lot slower than that of the risk-takers.
So here’s a question I have that’s just as relevant in the mathematics classroom: how do we get students to take risks? How do we un-train students from thinking they need to know exactly what to do before they can make an attempt?
(I was going to say that’s relevant to all subjects, but you probably want to hold back on the risks in, say, Chemistry pracs.)
Overall, I still don’t know how to judge my first effort at the subject. Did I do what I set out to do? Yes: we’ve now established links in the ICT curriculum all the way from Prep to VCE, my students produced some very impressive projects and even if some students weren’t as enthused as I’d hoped, they should have a better understanding of what VCE IT involves than students in the past. Am I perfectly happy with how it went? No: there are lots of areas I can see to improve.
That said, the day I ever say I’m perfectly happy with the way I teach a subject is the day I should resign.
* For some reason we refer to the subject as “ICT” up to Year 10, but it’s “IT” in VCE. I don’t know why. I always thought the “C” was redundant anyway.